Reviews for The Glass Wall: Why Mathematics Can Seem Difficult

The average rating for The Glass Wall: Why Mathematics Can Seem Difficult based on 2 reviews is 5 stars.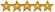

Review # 1 was written on 2011-07-04 00:00:00 Rue Jimbo Last year I did an elective in literacy and numeracy that I found breathtakingly interesting. To be honest, I had thought I would get much more out of the literacy half of the course and would more or less sleep though the numeracy section. Most other 'numeracy' subjects I have done in the past have tended to be about teaching people how to make estimates that are better than 'wild guesses' or to find ways to figure out how many basketballs might fit in a classroom. I don't believe all study should be immediately relevant to a student's life, but there does seem to be an assumption that if you are getting people to calculate just about anything then that it is both 'doing maths' and also that, in itself, will be 'doing them good'. This book is remarkable. Frank Smith sets out to look at just what it is that 'doing maths' means. One of the things we often think doing maths means is 'counting'. Kids get taught to count from a very young age. Essentially, this is a chant they learn. Now, most of us have learnt the Lord's Prayer as a chant too. I'm sure there have been people who have said this particular chant all of their lives but never really thought about what 'trespasses' might mean or why we might want to be forgiven them. It is also true that both a child and the Pope can recite the Lord's Prayer word perfectly, but that this is hardly proof that the child and the Pope have the same understanding of what they are saying. And so it goes with numbers too. One of the main problems is that numbers are remarkably abstract. As Smith says, we often think that when asked to explain what 3 means we have conclusively defined the number by holding up three pencils. However, only those who already know what three means could be satisfied with such a demonstration. And that seems to be true for virtually all demonstrations in maths - on a very deep level maths is tautological and that fact makes learning maths incredibly difficult until you 'know'. For Smith, maths is something that happens in the head of the person learning maths and so it is remarkably hard to help that person without first 'getting inside' their head, as it were. A task made infinitely harder on the 'teacher' as kids learn very early on to hide the fact they don't understand (or to hide what they think they understand) so as not to look stupid. In as far as this book is about learning how to help people who are struggling with maths to learn something of the subject, it approaches mathematics from the perspective of maths language. The kids that have the most trouble with mathematics - despite popular assumptions to the contrary - also tend to have problems with literacy. As my lecturer said last year - give primary school kids a numeracy test and if they fail you can virtually automatically enrol them in a reading recovery programme. The correlation drops off in high school - however, well over 50% of students who struggle with maths will also struggle with reading and writing even there. While some kids do finally catch on to reading - maths tends to work more on the Matthew principle, for those that have, more will be given; for those who have not, even that which they have will be taken from them. (Not quite sure why this review is turning out so 'religious' - but there you go) In one of the dialogues, Socrates complains about this new fangled idea of writing - his main concerns are that writing hinders people's memory (why bother memorising something when you can just look it up in a book?) and, unlike a real person, the voice in a book can only ever 'tell', it can never 'answer' - so you can't literally question a book, as it will merely repeat its same answer back at you forever. Not for the first time, Socrates is wrong here. It is highly likely that it was in being able to read and write that enabled Socrates to think (and yes, I mean literally think) in the way he did. This is a point made very clearly by Luria's work on the logical capacities of illiterates. Abstract thought needs something external to itself to fix on, it needs anchors. Being able to read allows 'fascination' (in the sense of fixed attention) and it allows this in a way that oral language is never capable of. Socrates' complaint that a book constantly tells you the same thing over and over is, in fact, its greatest strength. And being able to write is yet another guide to understanding. Often I have no idea what I think about the books I read until I have written one of these reviews - how much these reviews distort or in fact create my opinions on the books is very hard to say. We need a way to hear ourselves and to look at what we have said so as to be able to think about what we think - this is already my second draft of this review and I will read over it all again, at least once, before submitting it. As Smith points out, mathematics only became mathematics when it was written. Writing was a technology that allowed mathematics to be something more than mere counting. There is a desire in many people, and until reading this book, I included myself amongst 'those people', to find ways to use language to help kids understand mathematics. There is no question that the language demands of mathematics are remarkably high and that mathematics teachers are often blind to the linguistic difficulties, demands and confusions created by the very language that surrounds mathematics. Kids who learn English as a second language often struggle with prepositions (of, by, for, into - those deceptively short and simple looking words that are nearly impossible to define or explain - look them up in the dictionary sometime), and they are not alone (Bernstein showed working class kids tend to avoid 'for' and 'of' and 'by' - relational prepositions - and stuck with 'on' and 'in' - positional prepositions), but many problems in mathematics hinge on the preposition in the question for the student to have any idea of what the question is asking them to do. This situation is made worse by the fact that often the same preposition will mean you should do very different operations - 'by' for example can mean you are being asked to multiply, divide, add or even subtract (reduce the neutron flow by a factor of six and then increase the positron emissions by lots of twenty). This book could well have been called, the book of mathematical confusions. Not only is mathematical language dense (both lexically and grammatically), but language itself is also so imprecise that it smashes up against the abstract and idealised precision of mathematics. As Smith points out, there are no real linguistic definitions for such simple mathematical concepts as + or =. These seem completely obvious to us - that is, to those of us on the right side of the glass wall he talks about - but they are anything but obvious concepts and there are any number of reasons why kids might struggle to understand these ideas. They are, after all, ideas we would struggle to define in anything other than a tautology. There are many parts of this book that really brought me up short. For example, he discusses the problem of numbers and how well we are able to visualise them. If you were to glance at a group of objects and were then asked to guess how many there were - what do you think would be the maximum number of objects that you would be likely to identify without making a mistake? Before reading this book I probably would have said something like 7 or 8 - although part of me might have thought I was being a bit conservative. It turns out I was being grossly optimistic. Three or four is about our limit. One of my all time favourite quotes is by US Senator Everett Dirksen, 'A billion here, a billion there, and pretty soon you're talking real money'. But why is that funny? In part it is because most of us would be more than happy to call a single billion dollars 'real money' - but also it is partly because none of us can visualise a billion anyway, it is just too large a number. But the real problem is even worse than this - it is that none of us can visualise even a thousand. Or even a hundred. Mathematics is a trip into the unknown, a trip beyond our powers of imagination, even when we talk about numbers greater than five. We need mathematical tools to be able to do mathematics in any sense - but those tools only make sense from within the highly abstract and confusing world of mathematics itself. Language, in this arcane world, can both help us to acquire the tools we will need to do mathematics and hinder us in ways we, all too often, simply do not anticipate. Teaching kids to chant numbers (up to, say, thirty) doesn't mean that they understand those numbers, particularly not 'mathematically'. Part of the problem is with our very stupid number words. Words like eleven and twelve (which might better be called ten-one and ten-two) confuse kids to the 'mathematical truth' - that is, the mathematical pattern they should be learning - because the words themselves hide the placement system that makes our number system 'work'. And in hiding the placement system it stops kids from being able to 'think mathematically'. One of the 'daft' things about Roman Numerals is that the numbers for four, nine and all numbers like that on up through the system (40, 90), require you to stop reading left-to-right and suddenly start reading right-to-left. From VIII to IX - we literally need to switch to reading backwards. That is, before you can see that we have written 9 you need to see that we are talking about 'one before ten' - that is, read backwards. Before you laugh at those crazy Romans remember that all of our words for the numbers between 13 to 19 do exactly the same - they invert the placement order of those numbers. Asian languages tend not to do this - the words they use exactly match the placement system and this consistency has been used to partly explain why Asian kids tend to outperform non-Asian kids in maths. A further confusion is that thirteen sounds a hell of a lot like thirty, as does fourteen sound like forty and so on. If you wanted to come up with a system to make kids stumble it would have been hard to have come up with a better one. Smith points out that people learn best when the '3Cs' are met - People "expect consistency, coherence and consensus. By consistency, I mean we all expect the world to be the same tomorrow as it was today and yesterday . . . by coherence, I mean that we expect everything to hang together . . . by consensus, I mean we expect people to view the world and their experiences the same way we do." However, often mathematics does not allow these 3Cs to be met. My favourite example of expectations being shattered by mathematics is in multiplying by a fraction. It is an unspoken assumption that if you multiply two numbers together that you will get a bigger number - and this assumption generally holds true, right up until kids are confronted with fractions - then multiplying makes the answer smaller. Counting is also much more of a problem than we tend to assume. We like to say things like multiplication is just a form of addition - or division is counting the groups of one number contained in another. But these links to counting set kids up to fail. Many children learn they will get the right answer (at the very beginning of their journey into maths land, at least - often also where the journey ends for too many of them) if they 'count on'. What does 7 + 4 =? Well, starting at seven and counting on with their fingers they get eight, nine, ten, eleven - it equals eleven. Great! Now, what does 67 + 23 =? Not nearly so easy if you are counting on, but as simple as pie if you know your placement system - that is, if you are able to think mathematically and see patterns and number relationships. The two big lessons from this book are that maths is a subject that is about relationships (numbers only make sense in their relationship to other numbers and so learning maths is about learning the patterns numbers make) and also that mathematics is about finding easier ways to do things than 'counting'. Should kids be able to use calculators in class? Wrong question. The right question is, what tools can I use to help kids to think mathematically. If 'calculator' is a tool that helps them to think mathematically, then use it. If 'calculator' hinders them thinking mathematically, then don't. This really is a fascinating book. The main problem people face in learning to do maths is the difficulty teachers have in understanding why their students are not 'getting' what is, after all, blindingly obvious. And that is the tragedy of mathematics - what becomes blindingly obvious once you know is anything but up until you do.

Review # 2 was written on 2020-01-05 00:00:00 Andrew Stewart I have been on the wrong side of the glass wall as envisioned by this book. As a learner, mathematics made little sense to me. I couldn't remember the formulas, and I failed the subject in my final year of secondary school in 1981. Ironically, I remember my score of 45%. Now, having been a teacher for over 35 years, I find myself on the other side of that wall. I am gobsmacked that I only became aware of this title late last year in 2019 courtesy of a friend's recommendation. It was published in 2002! From the first page in this book and you are told that mathematics "isn't necessarily complicated or difficult, nor is it something that is accessible only to an intellectual elite. Mathematics should be open to everyone, provided no unnecessary obstacles are encountered" (p. vii). Frank Smith writes with such clarity about these complicated matters, the obstacles that can keep us behind the glass wall. In this book Smith is writing somewhat out of field, he is known for his work in relation to a whole language approach to the teaching of reading. Perhaps this is why he is able to reveal what makes mathematical understanding possible, and why it can be difficult. The obstacles to understanding mathematics are often rooted in language, how we talk about mathematics and how we write about it. These also reflect how we perceive and teach it. Rather than go into detail about the book's content, I'd like to share how I responded to reading it. Much of what I read, I read electronically, via my kindle, ipad or computer. However, I read an actual paper copy. And this changed my reading experience. With a pencil in hand, I felt like I was having a conversation with the author. I made notes, comments in the margin, smiley faces even, and successive exclamation marks when I agreed (and I rarely disagreed) with what he was saying. I was taken by his ability to express the complex relationship between language and mathematical operations, for instance, he writes, "Cells multiply by dividing" (p. 33). This lays bare the relationship between multiplication and division, but also the potential for confusion, which is brought to life in the pages of the book. Not understanding this relationship can keep a person on the wrong side of the glass wall denying mathematical understanding. The book reiterates how language can get in the way. The author at one point discusses what it means to develop number sense and how this is very different from how language develops. He explains that you never hear the phrase 'word sense' or the need to develop 'good word concepts'. That's not a thing. The Glass Wall emphasises the importance of developing understanding from inside mathematics, from the world of mathematics that minds have created over many, many years. The reader is reminded that "Piaget asserted that children have to invent or reinvent mathematics in order to learn it" (p. 15). I suspect that the world of school mathematics gives little time for such invention to occur. When talking about '4 eggs in the nest' or '3 pencils' to somebody, the idea of fourness and threeness is not obvious, unless you know 'four' and 'three'. As he says, "for as long as we regard something as obvious, we overlook the intelligence that went into making it self-evident" (p. 4). This is similar to how place-value is taught. When we tell the kids, 10 of these is 1 of those, then show them a number perhaps via a picture of an abacus, that, in itself, doesn't even reflect the pattern, then give them a worksheet to complete, what we have actually demonstrated is that we have overlooked the intelligence that makes place value self-evident to us, but perhaps incomprehensible to our students. Unsurprisingly, a couple of chapters are devoted to considering place-value, that is, how numbers larger than 9 are written and to the names given to such numbers. Smith writes "Numerical order is not found in the physical world, except when we put it there. There is no 'order' in the natural world because there are no numbers there. The notion of order … comes from our fertile brain" (p. 64). I had a conversation with a 16 year old in the course of my work and asked him how he might represent the number sixty-six. His first response of what to write is: '66'. I asked if he could show me another way, to which he responded by writing '60 + 6'. I then asked if he could show me without using numbers. He drew 66 tally marks clustered into chunks of 5 as a way of keeping track of a count of ones from 1 to 66. For him, 66 was a collection of 66 ones. A person aware of the place-value structure would also recognise 66 as 6 tens and 6 ones. When we fail to see the structure in our own mind, as this person had, we come up against the glass wall. The Glass Wall gives insight into the mathematics beyond counting, that of, calculating, measuring, the numbers that exist between numbers, and geometry, as well as some suggestions for getting beyond the glass wall of incomprehension. My only criticism is how memorising is treated. On the one hand, the author acknowledges that "Memory is organised on the basis of understanding. Everything we remember is connected to something else that we know…" (p. 116). I love this, yet, he then suggests a "minimal tool kit for basic mathematical memory" (p. 117) would have to include the number facts for addition and multiplication up to 10. If these facts are seen as separate entities to be committed to memory, then there are 200 discrete facts to 'learn'. There is an alternative to thinking this way, through teaching strategies that have a 'long shelf life'. For instance, consider 5 multiplied by 9 (5 nines) that can be thought of in terms of knowing 10 nines, 90, and so half of this is 45. I can apply this thinking to 5 multiplied by 23 (5 twenty-threes): thinking 10 twenty-threes, 230, half of which is 115. And this is just one of the strategies that might help a student get us beyond the glass wall. The book has given me a great deal to think about, especially in terms of the things we humans look for: "consistency, coherence and consensus" (p. 125), and what Smith has identified as the four essential conditions for learning mathematics. One of these conditions is 'time', that is, giving learners the 'gift of time' a phrase I first came across through a friend and colleague who introduced me to Richard Lavoie (see for instance his book, It's so much work to be your friend: Helping the child with learning disabilities find social success). Because, as the author of The Glass Wall says, "learning can't be forced … learned in a hurry" (p. 127). I highly recommend taking the time to read this book.